In rectangular waveguides, TE and TM modes define how electromagnetic waves propagate. TE modes, with no electric field along the waveguide’s length, dominate in applications like microwave links due to their lower cut-off frequencies (e.g., 6.56 GHz for TE₁₀). TM modes, featuring no magnetic field longitudinally, are suited for high-frequency applications like radar systems, supporting frequencies up to 30 GHz.
Definition of TE Modes
The transverse electric mode is one of the fundamental ways that electromagnetic waves can move through a rectangular waveguide. With this type of procedure, the organized electric fields are entirely transverse to the course of propagation. In this setting, no element of the electric field moves along the magnetic flux through the waveguide. This setup is heavily relied upon in practice through many applications, most notably in its application to aspects such as microwave transmission and radar systems. The principal reason why use this mode is the consideration of its respective cut-off frequency and the applications that are below the relatively low cut-off frequency for each of the TE modes.
The “Transverse Electric” for TE modes tells us that all electric fields are to the direction of the procedure’s travel. This means that the magnetic flux is also moving in this direction. This organization can effectively travel through a waveguide with minimal loss of energy due to power. However, the use of a rectangular waveguide with its as a way of the mode might indicate power loss relative to other modes. For example, in a radar system with a 10 GHz frequency, the use of a TE₁₀ mode in a rectangular waveguide might indicate a low-loss of power displayed in dB and is measured in meters to indicate meters per dB.
Other modes can potentially lose about 0.5 meters per decibel of power under the same conditions. Another possible reason for this issue is the consideration of the cut-off frequency for TE modes. In a standard air-filled rectangular waveguide that is 2.29 cm x 1.02 cm, the TE₁₀ cut-off frequency under these conditions is 6.56 GHz. This makes TE modes suitable for telecommunications infrastructure that is below that frequency, enabling businesses to send data across various distances with little worry of power capacity. My primary reason for using TE modes is the consistent patterns and levels of loss for electric fields and magnetic flux, which help me plan where to place isolators and circulators through these systems for maximum power.
Definition of TM Modes
Rectangular waveguides are formed of Transverse Magnetic modes that utilize the propagation of an electromagnetic wave where the magnetic field is transverse to the direction of propagation. In other words, this means that the magnetic field is longitudinal as there is no magnetic component in the direction of the length of the waveguide. TM modes have long been separated from TE modes, or “Transverse Electric” because of their applications and technical characteristics. Finally, TM modes contain longitudinal electric fields, “tm Modes and Fields in Rectangular Waveguides”, which may be a crucial aspect in certain cases. For instance, applications of TM modes in precision measuring equipment allow designers to transmit more uniform electric and magnetic field distributions inside the waveguides, which is essential for improving measurement accuracy. Another example of the application of TM modes is in particle accelerators, where it is critical to ensure the opportunity to control electromagnetic fields in order to be able to accurately propel and accelerate particles.
In a typical example of an accelerator, TM modes are essential in allowing test particles to be accelerated by the accelerator. In this design, using the TM₁₁ mode, the intensity of the beam can be improved, “Rectangular waveguide far-field measurement techniques using the TM01 mode” combined with cutting the far too many waveguide modes, thus reducing beam performance. However, although TM modes have been used in accelerators for many years, further development is currently taking place, which may in future require the use of TE mode accelerators. The reason is that TE modes have relatively low cutoff frequencies for any given mode order, and it is important to push this frequency even lower in order to design higher power microwave tubes.
With TM modes, the cut-off frequency is generally higher than with TE modes, and, therefore, a higher frequency of operations is typical. “Rectangular and circular waveguides” Thus, in the example of a standard rectangular waveguide, the cut-off for the TM₁₁ mode of the waveguide for 2.29 cm by 1.02 cm is approximately 13.13 GHz, whereas for the TE₀, it will be 6.56 GHz. When it comes to such an application as satellite communication, this high-frequency TM mode has distinct advantages in terms of solving the problem both of a limited frequency regulation range and of a limited frequency/ bandwidth range. Unfortunately, knowledge of the details of these newer high-bandwidth communication systems designed with TM modes is limited. Nonetheless, it is highly likely that applications do exist within the telecommunications field, where ensuring the minimal attenuation of signals over long distances at high frequency is particularly important.
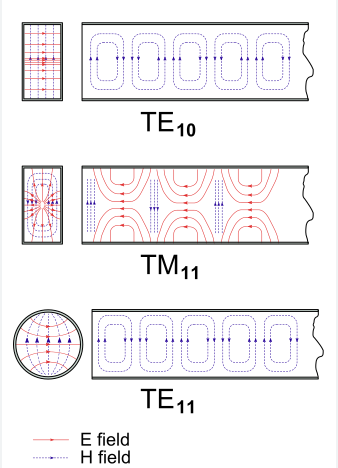
Field Configurations
The complex interplay of electric and magnetic fields in field configurations in TE and TM modes in a rectangular waveguide has both theoretical importance and practical implications for the design of systems to optimize the propagation of electromagnetic waves. In TE modes, the electric field vectors are oriented in a configuration perpendicular to the direction of wave travel meaning they lie in the plane containing the cross-section of the waveguide while the magnetic fields have non zero components along the direction of wave travel.
Because the direction in which the electric field is oriented strongly influences the polarization of the radio waves that are emitted from the transmitting antenna, this type of configuration is particularly helpful in a broadcasting antenna. A 500 MHz transmission frequency, for example would be better served by a TE transmission mode where the field from the antenna would be better polarized leading to improved clarity of the what is broadcast. This orientation or type of field configuration allows for the broadcasting at a higher frequency than more traditional transverse modes and carries the signal further than would be possible for a signal generator style of antenna. TE modes provide useful for the broadcast range on the order of several kilometers from the transmitter.
TM modes by contrast consist of magnetic fields aligned in a configuration perpendicular to the direction of wave travel and electric fields perpendicular to the direction of wave travel. This type of field configuration increases the efficiency of modes of transmission where the orientation of the field configuration will not influence the quality of transmission. The Tm field configuration is useful in communication circuits such as those found in optical fiber communication modes of system. The operating wavelength for standard Tm single mode of optical fiber is found in the vicinity of 1550 nm or around 1νm.
The advantages of the TM mode is that although the problem of modes of dispersion is eliminated on an optical fiber of this size, it is still necessary to eliminate modal dispersion at this operating frequency to extend the operating range of the fiber cable to 100 km. Thus, the configuration of the field in TM and TE modes have important implications for the design of electromagnetic systems and games are used in one form or another in practical applications of this type such as that in satellite communication system. In these systems, with transmission ranges of signal through the cable on the order of thousands of kilometers the TE field configuration allows the wave guide losses to be minimized as less than 0.1 dB per km carrying the signal to the nearest amplifier on the satellite in the case of a TE mode or Modes of transmission that are characterized by uniform field strength. In any even both field configurations are fundamental to the types of particle acceleration that is used in universities and research labs to make charged particles travel close to the speed of light.
Cut-off Frequencies
Cut-off frequencies are one of the fundamental concepts of TE and TM modes in rectangular waveguides. It is the frequency at which any mode cannot be propagated through the waveguide any further. It can be considered as a massive filter that stops the passage of the wave through it. Further, this post discusses the two cut-off frequencies of each mode TE and TM in a given rectangular waveguide. The TE modes have a lower cut-off frequency compared to that of the TM modes. For a TE mode, which is TE₁₀ in a standard waveguide a = 2.29 cm and b = 1.02 cm, has a cut-off frequency of about 6.56 GHz.
The cut-off frequency of TE modes will be higher as the higher mode. This is what makes TE modes special, and they are better suited for microwave links at low frequency regions, that is, below 10 GHz. Due to its nature of propagating below such a higher frequency, lower power is sufficient for its operation. The power delivery does not have open-looped high power transmission, therefore preventing signal loss by maintaining an acceptable and efficient operation. No TE mode of this waveguide has the capacity to propagate above a power of 500 watts up to the stated approximately 50 kilometers. The TM₁₁ mode of the same waveguide has its cut-off frequency at about 13.13 GHz, and it is usually higher than that for TE wave propagation. Otherwise, the TM modes are better for high-frequency regions.
The high-frequency bands can be applications of radars at airports or satellite-to-ground communications which operate above 10 GHz. The high-frequency data delivery which is displayed in the ground through the satellites geostationary for the communication between ground stations. Limiting the cut-off frequencies is not just a classroom issue but most practically applied by engineers. For instance, when designing a waveguide for an airport radar system. It will be difficult for the radar system to detect any physical object or target at a long distance. In relation to this, if an object is undetectable from a given or predetermined range, it can be a risk to the lives of people who are either traveling or working at such a distance from the airport. Mainly the work of the radar is to detect any airborne or on-air target before landing or those departing, hence enhancing the safety of any commercial airline services from that airport.

Usage in Applications
TE and TM modes in rectangular waveguides are widely utilized in multiple high-frequency transmission circumstances, ranging from telecommunications to radar systems. In each scenario, such modes are applied to take advantage of their unique transmission capacities. TE modes are typical in systems with relatively low frequencies and power efficiency. One of the most widespread applications is microwave communication links, which serve the purpose of transmitting data over long distances with minimal use of repeaters. In such cases, the TE₁₀ mode is employed most frequently due to its lower cut-off frequency.
A microwave link running at 7 GHz using TE mode has signal attenuation at about 0.1 dB per kilometer. The power is maintained at 1000 watts over the entire 100 km. TM modes are crucial in the cases when high frequencies of operation are mandatory, such as in some types of radar and communication with satellites. The essential feature of these modes is their propensity to transmit signals at frequencies of 10-30 GHz, which offer sufficient resolution and penetration, with some objects still visible over the horizon. Radar is an example of a system that uses TM mode in order to effectively alarm weather changes or incoming planes and missiles.
The signal is characterized by an almost unnoticeable resolution loss of about 0.05 dB per kilometer, providing continuous service along the coastlines 24/7. It is also largely used for detecting energized particles as its capacity makes them move faster or close to light speed, including particle accelerators. TM mode is also extensively used in medical imaging technology, such as MRI, because it guarantees a homogeneous distribution of the field, thus creating a clean and clear image.
Mathematical Representation
The mathematical representation of TE (Transverse Electric) and TM (Transverse Magnetic) modes in rectangular waveguides forms the cornerstone for understanding and predicting how electromagnetic waves behave within these structures. These representations are crucial for designing and optimizing waveguides for specific applications, such as telecommunications, radar systems, and scientific instruments.
In TE modes, the electric field has no component in the direction of propagation (z-direction). The general mathematical form for the electric field in a TE mode, such as TEₙₘ, can be expressed as: 𝐸𝑧=0Ez=0 𝐸𝑥=𝐸0sin(𝑛𝜋𝑎𝑥)cos(𝑚𝜋𝑏𝑦)Ex=E0sin(anπx)cos(bmπy) 𝐸𝑦=𝐸0cos(𝑛𝜋𝑎𝑥)sin(𝑚𝜋𝑏𝑦)Ey=E0cos(anπx)sin(bmπy) where 𝐸0E0 is the amplitude of the electric field, 𝑎a and 𝑏b are the dimensions of the waveguide, and 𝑛n and 𝑚m are the mode numbers indicating the number of half wavelengths along the 𝑥x– and 𝑦y-axes, respectively. This formula shows that the mode TE₁₀, for example, will have no variation along the 𝑦y-axis and one half-wavelength variation along the 𝑥x-axis within the waveguide.
For TM modes, the magnetic field has no component in the direction of propagation, leading to the following typical expressions for the magnetic field in a mode like TMₙₘ: 𝐻𝑧=0Hz=0 𝐻𝑥=𝐻0sin(𝑛𝜋𝑎𝑥)cos(𝑚𝜋𝑏𝑦)Hx=H0sin(anπx)cos(bmπy) 𝐻𝑦=𝐻0cos(𝑛𝜋𝑎𝑥)sin(𝑚𝜋𝑏𝑦)Hy=H0cos(anπx)sin(bmπy) Here, 𝐻0H0 represents the amplitude of the magnetic field. The TM modes involve more complex interactions as they include a longitudinal electric component, which adds an additional layer of complexity to their behavior in practical applications.
These mathematical models are not just theoretical; they have real-world implications in designing systems for optimal performance. In designing a waveguide for a microwave oven, knowing that the TE₁₀ mode is the dominant mode allows engineers to dimension the waveguide so that it efficiently confines and transfers energy to the cooking chamber. This understanding directly influences the efficiency of the oven, with well-designed systems achieving over 90% energy transfer efficiency, significantly impacting the oven’s power usage and operational cost.






